Technical Articles

Shortcut on the Way to Freeform Optics

Download

Shortcut on the Way to Freeform Optics

Published in: Kunststoffe International 12/2013, page 58-61

Authors: Lars Dick (Jenoptik Polymer Systems GmbH) und Laura Florez

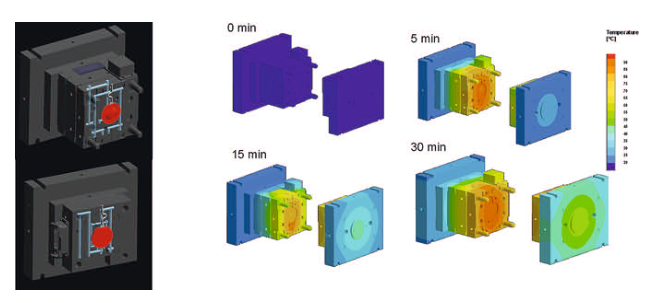
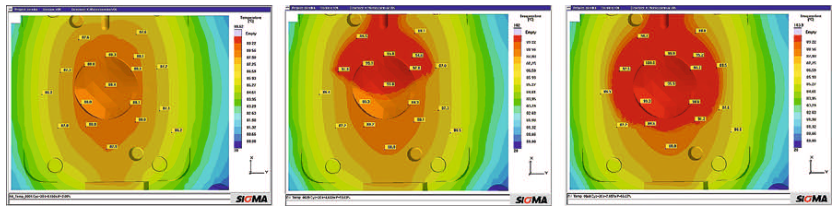
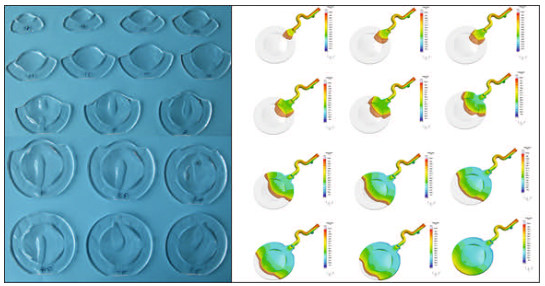
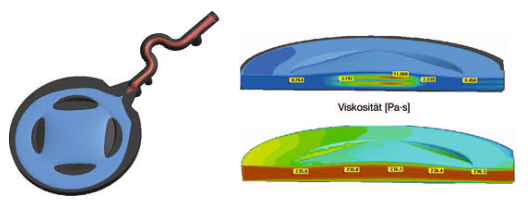
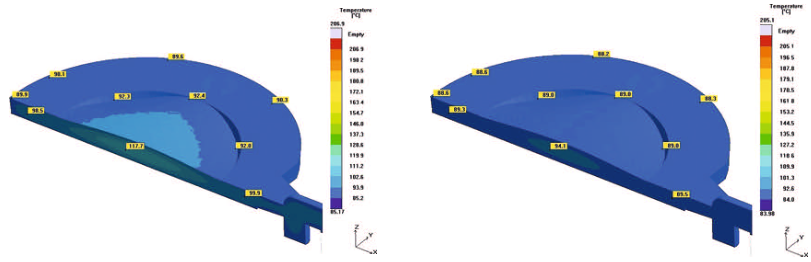
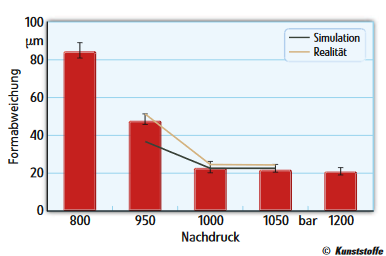
Virtual Mold Trials. The manufacture of plastic optical components usually involves a highly iterative process during development of the molded part and mold construction. Reason for this is the uncertainty associated with the injection molding behavior. The case example from a research project demonstrates how a virtual mold trial can replace this stepwise approach, which also results in considerable cost reductions.

Optical components manufacturing is one of the most demanding tasks for injection molding. Precise dimensions, tight tolerances, and the persistent demand for faultless and reproducible parts represent the operational framework in this industry. Driven by increasing miniaturization and more functionality in new device generations in the past years, the industry has shown a strongly increasing demand for high-precision injection molded optical components. Analogous to the innovative impulses for new, more compact equipment system triggered in recent years through the functional options of aspheres, new perspectives for future system innovations are presently emerging through the use of application-specific freeform optics. This nomenclature summarizes refracting and reflecting surfaces that clearly differ from symmetric and aspheric geometries.

However, due to inadequate availability of technical basics, today's demand for plastic types for freeform optics can only be met at uneconomic expense. Within the scope of the FREE project – the acronym stands for “precision freeform optics” – Jenoptik Polymer Systems GmbH in Triptis, Germany, was faced with the challenge of producing symmetric, non-rotation freeform optics from high-performance plastic materials. Hereby, the aim was to achieve a precision of 0.5 µm. In order to penetrate this lucrative business, in which polymerbased precision optics with symmetric, non-rotation freeform profiles play a key role, this project was to create the required basic competences.

Figure 1: SIGMASOFT® Virtual Molding examines all the information on mold tempering. This permits the temperature distribution at every point in the component and mold to be represented for different points in time during several cycles. Shown at left are the tempering channels on both sides of the mold

Matching the Process for Freeform Surfaces
For optic components made of plastic, manufacturers have a basic choice between replicative methods of injection or compression molding, and material removal methods of ultra-precision diamond machining. While ultra-precision machining methods are typically used for small to medium-sized batches – i.e. characteristic for prototyping tasks –, replicative molding methods are only employed in series production because of the high
preparatory costs. These costs mainly include the complex production of the master molds, which requires a considerable advance investment, and, due to the only vague knowledge of the injection molding process, frequently involve several iterative loops.

For non-symmetric freeform surface profiles the machined implementation of the complex geometry in a ductile mold material with the necessary optical quality as well as the necessary measurement technology are very complex.The investigation and determination of process conditions during injection molding for these precise requirements is a so far unsolved task.

In this case, the simulation tool “Sigmasoft Virtual Molding” (Sigma Engineering GmbH in Aachen, Germany) was
used to analyze the influence of different process parameters on component quality. For every step in the calculations, the molded parts and the replication mold are closely coupled. Calculations for form filling are based on the complete 3-D Navier Stokes flow equations. These are followed by a true 3-D calculation of post-pressure and cooling phase, plus component shrinkage and deformation until room temperature is reached.

After an initial thermodynamic consideration, a mold heat-up time is determined, followed by a count of the cycles
required until thermal equilibrium is reached, which is essential for an expedient replication process. Various key aspects are examined in the following simulations of the filling process:

Flow fields,
pressure and temperature distribution in the melt at different points in time,
development of the flow front during mold filling,
possible locations of weld lines and air pockets,
representation of mold filling and flow phenomena behind the flow front by means of (massless) tracer particles.

Following the simulated filling phase, the
cooling and post-pressure phase is examined, whereby temperature distribution during the cooling phase is in the
forefront, in order to determine an optimum residual cooling time. Subsequently, shrinkage and deformation in
all spatial directions are determined and
displayed.

Fig. 2. Energy introduced by the hot plastic melt during the injection phase causes a temperature increase from 88.4 to 95.5 °C in the freeform surface region of the mold

Thermal Analysis of the Mold
At first, a thermal simulation is used to establish for how long hot water at 90 °C must flow through the mold's tempering channels until the target temperature or a thermodynamic equilibrium is practically reached (Fig. 1). Initially, the mold absorbs a great amount of energy. After about 10 minutes,the energy input is considerably lower, but a stationary condition has not yet been reached. After a 30-minute heat-up time with 90°C hot water, a homogeneous temperature distribution between 86.5 and 86.8 °C is noticeable in the cavity. Therefore, this
time should be allowed in the practical tests (Table) before the first molded part is produced.

On the other hand, the manufactured parts generate a cyclic thermal input to the overall thermodynamic system, in accordance with the established balance equation:
Qmelt + Qtempering = Qcomponent + Qradiation

Accordingly, the amount of energy introduced by the melt (Fig. 2) and the mold's tempering channels equals the sum of energy removed by the molded part and through heat radiation of the mold. If the sum of all these energy flows does no longer changes, the system exhibits the same energetic state before and after the cycle. Consequently, it has reached a quasi-stationary state, and production of good parts can commence. For example,
if it takes 30 cycles to reach this state of equilibrium, the first 30 parts of every test series must be seen as scrap. Useful measurements can only be made from the 31st part onward.

Fig. 3. The simulated filling process correlates well with the practical results

Analysis of Filling Behavior
The filling simulation is followed by a thermal investigation of the replication process. Hereby, possible jetting, flow
lines as well as air pockets can be detected. The homogeneous filling process determined by the simulation correlates closely with the results of the injection tests (Fig. 3). For the practical comparison, a filling study was conducted, whereby only a defined portion of material is injected into the mold, which is not enough for complete mold filling. For the first part in the diagram, 17 cm3 of shot volume remain in the dosing unit, and only 3.5 cm3 for the last part.

The simulation shows how melt temperature changes during the filling phase. Here, one sees clearly that some surface areas of the component near the gate cool down significantly,whereby temperatures of 130 °C are reached. Because of the long filling time, a temperature difference results in the component at the end of the filling phase (Title figure). An explanation for this can also be provided by the simulation, where the flow conditions behind
the flow front are shown by so-called tracer particles (massless particles). It shows clearly that in several areas near the gate the melt flow is slower and cools down more quickly than in areas in which the plastic is still subjected to high shearing forces or hot melt continues to enter the mold.

Fig. 4. In the post-pressure phase effective pressure is reduced with increasing distance from the gate

Post-pressure and Freezing Behavior
Noticeable in the simulated pressure distribution of the component is that the adjusted post-pressure of 1,000 bar only exists on the nozzle side of the gate. With increasing distance from the gate, the effective pressure drops. At the beginning of the post-pressure phase, 968 bar are measured in the component at a distance from the gate, and 975 bar near the gate. At the end of the post-pressure time, pressure distant from the gate is only 600 bar, and 622 bar near the gate (Fig. 4). In a next step, component freezing can be simulated (Fig. 5). This is highly relevant, as this simulation provides reliable findings regarding post-pressure time and residual cooling time. A constant post-pressure only has a positive effect on molding accuracy as long as a liquid core can be maintained between gate and component.

Frozen areas are shown transparent. Red areas are still molten and are are affected by post-pressure. Blue areas are still molten, but can no longer be supplied with post-pressure. Here, only the attained pressure is relevant for compensating the plastic’s volume shrinkage. The component can be affected by dwell pressure up to some 90 % of frozen volume. After 30 s of post-pressure time, the necessary connection to the lens is frozen, so that a longer post-pressure time for increased molding accuracy makes no sense (Fig. 4, right). Therefore, the originally defined post-pressure time was increased from 20 to 30 s in the course of the investigations.

In the post-pressure phase, only very low shear values and also lower temperatures occur. This leads to a strong increase in viscosity during the post-pressure phase. In turn, high viscosity means that it becomes more difficult to press melt into the mold. What does the simulation reveal about cooling time? For this, temperature across the component cross-section was initially analyzed 100 s after the filling process (value calculated with a Fourier
transform). As shown in Figure 6, the central region is almost at 118 °C, and therefore still above the glass transition temperature. After the defined residual cooling time of 180 s, and a dwell time of 30 s (cooling time = 210 s), the temperature in the central region has dropped to about 94 °C, and therefore below the Vicat softening temperature (103 °C), while the border regions of the freeform surface are at 89 °C, i.e. almost at cavity temperature. 30 minutes after demolding, the component’s core temperature has reached 25.3 °C, i.e. practically room temperature.

Finally, deformation is examined. Across the diameter (44 mm) the surface shrinks almost uniformly by 180 µm. This
equals a shrinkage of 0.41 %,which is very close to the measured shrinkage of 0.3 %. Also in the comparison of mean deformation as a function of dwell pressure, the measured and predicted values correlate very well (Fig. 7).

Fig. 5. Simulated freezing process of the freeform optics, with 30 s post-pressure time, and the viscosity at beginning and end of post-pressure time. The predicted freezing behavior permits conclusions regarding post-pressure time and residual cooling time. Shown at right is viscosity at the beginning (top) and end (bottom) of the post-pressure phase

Fig. 6. Simulated temperature distribution in the component with cooling times of 100 s and 210 s

Summary
Today, the manufacture of optical components often involves complex iteration loops before the production starts. Sigmasoft Virtual Molding can predict the interactions between process parameters and component quality. This permits development time and costs to be saved. Moreover, the cause for undesirable defects can be detected and eliminated at an early stage.

Fig. 7. Calculations for shrinkage and deformation investigate the influence of process parameters on component quality during several cycles. The predicted effect of post-pressure on mean component deformation coincides closely with reality

Properly Cooled by Design

Download

Process-oriented Injection Molding Simulation. Besides the part geometry, 3-D injection molding simulation now also takes into account other factors promoting the efficient design of injection molds and assisting process control during production. A case study makes clear the benefit of process-oriented simulation with the example of a decision in favor of a cooling concept tailored to requirements.

Kunststoffe International 12/2011, page 24-26

Currently, there are only a few plastics articles that are still developed without injection molding simulation, which usually focuses on predicting the filling pattern and part warpage. Nevertheless, the use of this software tool has reached a stagnation level. In the 20 years and more since injection molding simulation became established in the plastics industry, a general opinion about the benefits and reliability of simulation has become established in the industry. If we look more closely at this judgment, we can often see that it is the result of experience with conventional or 2.5D Hele Shaw-based approaches to simulation.

However, the state of the art has really moved on significantly from this. Besides plastics articles, all mold components, such as platens, cores, slides and ejectors, with their physical material properties, can now be modeled completely three-dimensionally, and therefore physically correctly, in the injection molding simulation. On this basis, the entire injection molding process can be described in detail, and its quality and energy efficiency assessed. These comprehensive possibilities are based on the three-dimensionally coupled simulation of transient heat transfer between the melt, mold and cooling system, and therefore a physically precise description of the interaction between the rheology of the polymer and the mold’s thermal performance.

With this approach, known as “process oriented”, an injection molding simulation using Sigmasoft (supplier: Sigma Engineering GmbH, Aachen, Germany) can register all the relevant influencing factors, such as the article, mold, hot runner and process control. This gives the user two advantages – increased accuracy and validity, together with a broader scope of applications.

Fig. 1. Filling study of a housing design of PA66-GF50 (left). The process simulation, at the end of the filling phase in the 22nd production cycle in this case, illustrates the 3-D temperature distribution through the entire mold (right)

Multicycle Simulation: Closer to Reality

Once the entire mold can be modeled in the simulation, it no longer makes sense just to simulate one injection molding cycle. The potential of process-oriented simulation is rather to use the simulation of multiple sequential injection molding cycles to determine the thermal-rheological state of the mold during actual production. To achieve these production conditions, heating of the mold through multiple complete cycles (mold closing, injection, cooling, mold opening, demolding, mold closing …) is simulated, and the energy input and removal of the different components is simulated appropriately.

This makes it possible to determine, for example, how many starting cycles are used for the production start of a mold, or after a production interruption, in order to reach a steady state again – a simple approach for minimizing rejects. This method also illustrates the actual performance of the chosen cooling system, which can now be assessed in terms of cost efficiency, article quality and energy efficiency, as is shown by the following practical example.

Fig. 2: Practical example: A process simulation allows the efficiency of alternative cooling concepts to be compared (figures: Sigma Engineering GmbH)

Practical Design of the Cooling System

A typical housing application of PA66-GF50 (Fig.1, left) illustrates the potential of process-oriented injection molding simulation with the selection of a cooling concept. The result of the simulation of a standard cooling concept is shown by the cutaway view of the entire mold, and, in colors, by the local temperature distribution at the end of the filling phase in the 22nd production cycle. The heat exchange between the article, mold components and cooling system can be clearly seen, as can the temperature profile in the mold resulting from the transient heat transfer (Fig.1, right).

After approval of the part design, the development team was confronted with the question of whether a standard cooling system is sufficient for efficient cooling of the part, or whether conformal cooling improves the cycle time, part quality and energy efficiency. There are currently many conformal cooling systems on the market, and, without process-oriented injection molding simulation, it is difficult to quantify the advantages in advance.

In a first step, two alternative cooling concepts were therefore drafted and compared (Fig.2). Process simulation with Sigmasoft was carried out to analyze and evaluate the performance of the respective mold design, and provide facts to support the decision for the most suitable cooling system.

To assess the cooling systems, 15 successive injection molding cycles for the two models were simulated. A comparison of the temperatures at the stationary mold half shortly before the end of the filling phase makes it clear that the mandrel-shaped mold core in the foreground is only poorly cooled by the simple cooling concept (Fig.3, left). Here, the temperatures at the core surface are between 130 °C and about 142 °C, which prolongs the solidification time at these points, and therefore the cycle time as a whole. The solution with conformal cooling (Fig.3, right), on the other hand, shows a more homogeneous temperature distribution over the entire mold cavity with temperatures on the core surface of about 95 °C, which reduces the solidification time by up to 23 % (Fig.4).

The differences in the part solidification are illustrated by colored regions, which indicate hotspots in the part (Fig.5, top). At these points, the polymer stays fluid for longer than at the surface, and in particular longer than in adjacent part regions. In the comparison of the two results, the significantly more voluminous hotspots that occur in the mold with standard cooling are immediately conspicuous. With the simple cooling concept, liquid regions can actually still be found after 2s cooling time.

In this application, conformal cooling reduces the cycle time as well as the warpage (Fig.5, below). Although for a polymer reinforced with 50 % glass fibers, the fiber orientation clearly dominates the warpage, the differences in the mold temperatures still show an effect. More homogeneous cooling reduces internal tensions in the region of the core, and improves the warpage behavior at these points by up to 13 %.

Fig. 3: The evaluation at the stationary mold half at the end of the filling phase after 15 production cycles shows a more homogenous temperature distribution throughout the mold cavity for conformal cooling

Evaluation of the Energy Efficiency

Besides the cycle time and article quality, energy efficiency is also increasingly becoming a determining issue. If the heat balances of the two cooling concepts are compared, it can be read from the curves how much heat (in kW) per cycle is exchanged in the mold (Fig.6). The melt introduces energy into the mold; the mold platens lose energy to the surroundings, and the cooling systems are required to bring the entire energy balance into equilibrium by absorbing and emitting energy. Over time (multiple cycles), the absorbed energy becomes smaller. If this decrease stabilizes, the mold has reached the quasi-stationary thermal state, and production can begin.

The curve profile proves that the energy requirement for heating the mold with the simple cooling concept is greater than with conformal cooling. Conformal cooling achieves an asymptotic energy absorption at a comparatively early stage – after about eleven cycles – while simple cooling is still not stable even after 15 cycles. Conformal cooling thereby consumes less energy overall in this case, and production can start earlier.

Fig. 4. Conformal cooling reduces the solidification time by almost a quarter compared to the standard system

Summary

Injection molding simulation is no longer only limited to the part development phase, but can also act as an effective aid in the subsequent development phases, and provide detailed information for supporting many decision-making processes during mold development, trial moldings, identification of process windows and troubleshooting during production. The application example clearly shows how conformal cooling has been quantitatively analyzed based on an integrated consideration of the results, and it can be assessed at an early stage whether the extra costs are worthwhile.

Fig. 5. The part quality is affected by the choice of cooling concept. Simple cooling shows more hotspots (top), recognizable by the colored areas, and worse warpage (bottom) than conformal cooling